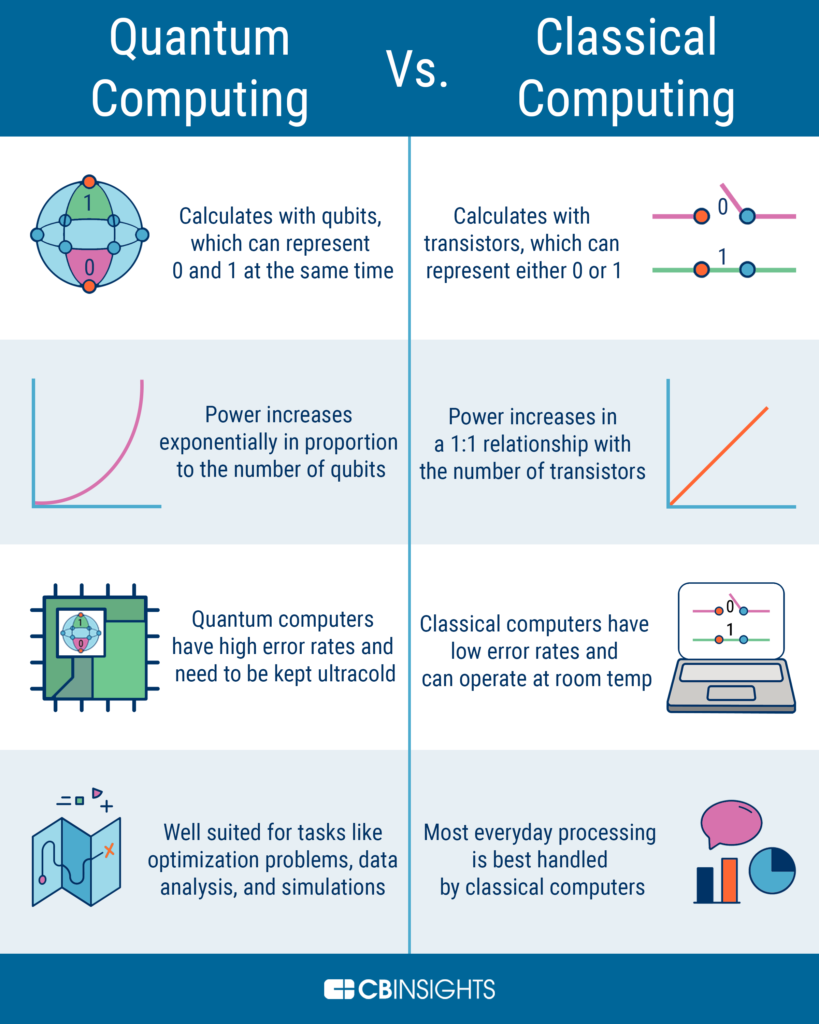
Quantum computing is a rapidly growing field with a lot of potential to revolutionize the way we process information. However, the subject can be quite complex and intimidating to those who are unfamiliar with the underlying principles. One of the fundamental building blocks of a quantum computer is the quantum gate, which is a unitary operation that acts on a quantum state. These gates are represented by matrices, but what do the columns of a quantum gate matrix represent?
The columns of a quantum gate matrix are a crucial component of understanding how quantum gates work. They represent the behavior of the gate on the basis states of the quantum system. Basis states are the simplest possible quantum states that can exist for a particular quantum system, and they form the basis for constructing more complex states. By understanding how the columns of a quantum gate matrix transform the basis states of a quantum system, we can gain insight into the behavior of the gate as a whole. This knowledge is essential for designing and implementing quantum algorithms, which are the key to unlocking the power of quantum computing.

What do the columns of a quantum gate matrix represent?
Quantum gate matrices are powerful tools used to describe quantum operations in physical systems. Each column of the matrix represents a quantum gate that can be applied to a system, and the columns together form a quantum circuit. By understanding the columns of a quantum gate matrix, one can better understand the behavior of a quantum system and the effects of applying different quantum gates.
How a Quantum Gate Matrix Works
A quantum gate matrix is a two-dimensional array of complex numbers that represent a unitary transformation. The columns of the matrix correspond to a set of quantum gates that can be applied to a quantum system. Each of these quantum gates has a specific effect on the system, such as entangling two qubits or transforming a single qubit from one state to another.
These quantum gates can be combined in different ways to form a quantum circuit. The columns of the matrix can be seen as the steps of this circuit, with each column representing a specific quantum gate. By understanding how each gate affects the system, one can construct a quantum circuit that performs a desired operation.
Quantum Gate Representations
The columns of a quantum gate matrix can be represented in a variety of ways. One common representation is a unitary matrix, which describes the transformation of a quantum system from one state to another. This matrix is composed of complex numbers, with each column representing a quantum gate and each row representing the transformation of a single qubit.
Another representation is the Pauli matrix, which describes the action of a single quantum gate on a system. This matrix is composed of the Pauli matrices, which are a set of special matrices that describe the action of a quantum gate on a quantum system. By understanding the Pauli matrices, one can better understand how each quantum gate affects a system.
Conclusion
The columns of a quantum gate matrix represent a set of quantum gates that can be applied to a quantum system. Each column corresponds to a specific quantum gate, and the columns together form a quantum circuit. Quantum gate matrices can be represented in a variety of ways, such as a unitary matrix or a Pauli matrix. By understanding the columns of a quantum gate matrix, one can better understand the behavior of a quantum system and the effects of applying different quantum gates.
Frequently Asked Questions
This article covers questions about the columns of a quantum gate matrix and how they are used. It provides an overview of the different types of matrices and their functions.
What is a quantum gate matrix?
A quantum gate matrix is a mathematical representation of certain quantum operations. It is used to describe the behaviour of a quantum system, and is essentially a matrix that contains information about the system’s state and how it will change over time. Quantum gate matrices are used to perform operations on qubits, which are the basic unit of information in a quantum system.
Each element in the matrix is associated with a quantum gate, which is a specific type of operation that can be performed on the system. The columns of the matrix represent the inputs to the gate, while the rows represent the outputs. In other words, the columns of the matrix represent the qubits that will be manipulated by the gate, while the rows represent the resulting states of the qubits.
What are the different types of quantum gate matrices?
There are two main types of quantum gate matrices: single qubit gates and two-qubit gates. Single qubit gates are used to manipulate a single qubit, while two-qubit gates are used to manipulate two qubits at the same time. Single qubit gate matrices are typically 2×2 matrices, while two-qubit gate matrices are typically 4×4 matrices.
In addition to single qubit and two-qubit gate matrices, there are also “controlled” gate matrices. These are matrices that contain more than one qubit and are used to perform operations on multiple qubits at the same time. For example, a controlled-NOT gate matrix contains two qubits and is used to flip the state of one qubit based on the state of the other qubit.
What is the purpose of a quantum gate matrix?
The purpose of a quantum gate matrix is to describe how a quantum system will behave when a certain operation is performed. By manipulating the elements of the matrix, it is possible to create a wide range of different quantum operations. This makes quantum gate matrices a powerful tool for exploring the behavior of quantum systems.
In addition, quantum gate matrices can be used to program quantum computers. By encoding the desired operation into the matrix, it is possible to execute the operation on a quantum computer. This makes quantum gate matrices an essential tool for quantum computing research.
How are quantum gate matrices used in quantum computing?
Quantum gate matrices are used in quantum computing to program quantum computers. By encoding an operation into a quantum gate matrix, it is possible to execute the operation on a quantum computer. The quantum computer will then use the matrix to determine how to manipulate the qubits in order to produce the desired outcome.
In addition, quantum gate matrices can be used to analyze the behavior of quantum systems. By manipulating the elements of the matrix, it is possible to create a wide range of different quantum operations. This allows researchers to explore the behavior of quantum systems and gain a better understanding of how they work.
What is the difference between a quantum gate matrix and a classical matrix?
The main difference between a quantum gate matrix and a classical matrix is that a quantum gate matrix contains information about the behavior of a quantum system, while a classical matrix only contains information about the behavior of a classical system. A classical matrix can contain information about the state of a single qubit or multiple qubits, but it cannot contain information about the behavior of a quantum system.
In addition, quantum gate matrices are used to perform operations on qubits, while classical matrices are used to perform operations on classical data. This makes quantum gate matrices an essential tool for exploring the behavior of quantum systems and programming quantum computers.

Rows and columns in quantum-gate matrices read the same – why?
In conclusion, the columns of a quantum gate matrix represent the effect of the gate on the basis states of a quantum system. Each column corresponds to a different input state, and the entries in the column specify the output state that results from applying the gate to that input state. Understanding the meaning of the columns is essential for designing and implementing quantum algorithms, as well as interpreting the results of quantum experiments.
As quantum computing continues to advance, the importance of understanding quantum gate matrices will only increase. With more and more applications of quantum technology, from cryptography to drug discovery, it is crucial for researchers and developers to have a deep understanding of the mathematical foundations of quantum computing. By grasping the significance of the columns of a quantum gate matrix, we can unlock the full potential of quantum computing and make groundbreaking strides in science and technology.