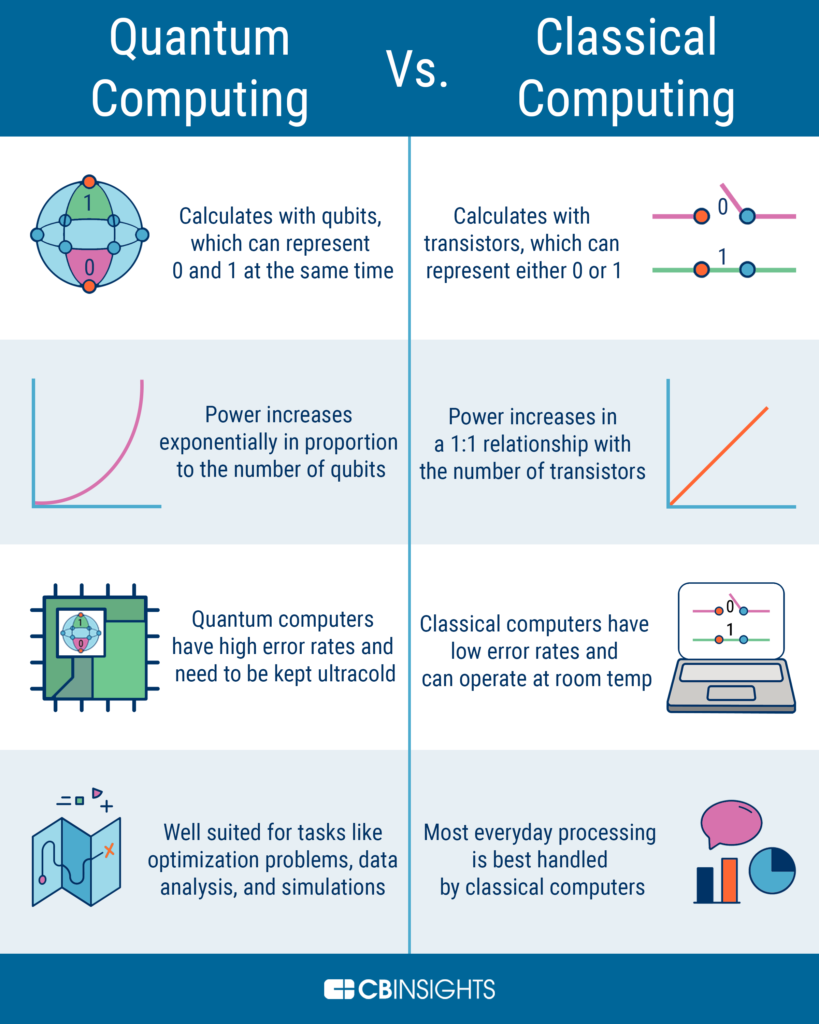
Quantum computing has become a buzzword in the technology industry in recent years. With its promise of exponentially faster computations, it has the potential to revolutionize industries ranging from finance to medicine. At the heart of quantum computing lie quantum gates, which manipulate the quantum state of qubits (quantum bits) to perform computations. However, the number of possible quantum gate matrices is a topic that often confounds many researchers and enthusiasts.
So, how many quantum gate matrices are there? This question has puzzled the minds of many experts in the field of quantum computing. While the answer may seem simple at first, the reality is far more complex. In this article, we will explore the different types of quantum gates and the number of possible quantum gate matrices that can be created from them. Join me as we delve into the fascinating world of quantum computing and explore the limits of what is possible in this exciting field.

How Many Quantum Gate Matrices Are There?
Quantum gate matrices are mathematical objects used to describe how quantum systems evolve over time. They are a key tool for understanding the behavior of quantum systems and designing quantum algorithms and protocols. In this article, we will explore the number of quantum gate matrices that exist and the different types of quantum gates.
Types of Quantum Gates
There are three main types of quantum gates: single-qubit gates, two-qubit gates, and multi-qubit gates. Single-qubit gates act on a single qubit and can be used to rotate the qubit’s state or to apply a phase shift. Two-qubit gates act on two qubits and are used to entangle the two qubits and create correlations between them. Multi-qubit gates act on more than two qubits and can be used to perform operations on larger systems.
Number of Quantum Gate Matrices
The number of quantum gate matrices is determined by the number of qubits in the system and the type of gate used. For single-qubit gates, there are four possible matrices, each of which is a 2×2 complex matrix. For two-qubit gates, there are sixteen possible matrices, each of which is a 4×4 complex matrix. For multi-qubit gates, the number of matrices increases exponentially with the number of qubits, so for a three-qubit system there are sixty-four possible matrices, each of which is a 8×8 complex matrix.
Quantum Gate Matrices in Practice
Quantum gate matrices are used in a wide range of applications, from quantum computing to quantum cryptography. They are used to construct quantum circuits, which are the building blocks of quantum algorithms. They are also used to simulate the behavior of quantum systems, allowing researchers to explore the effects of different types of gates and to design new quantum algorithms.
Quantum Computing
Quantum gate matrices are essential for quantum computing. They are used to construct quantum circuits, which are used to run quantum algorithms. By performing calculations on these quantum circuits, it is possible to solve problems that are too complex for classical computers.
Quantum Cryptography
Quantum gate matrices are also used in quantum cryptography. They are used to construct quantum circuits that can be used to securely send messages. Quantum cryptography relies on the principles of quantum mechanics to ensure that messages are secure and cannot be intercepted by an eavesdropper.
Frequently Asked Questions
What is a Quantum Gate Matrix? A Quantum Gate Matrix is a matrix that is used to represent a quantum gate in a quantum circuit. The quantum gate matrix is a unitary matrix, which means it preserves the inner product of the input and output states. A quantum gate matrix can be used to define a quantum operation on a set of qubits.
How Many Quantum Gate Matrices Are There?
There is no single answer to this question, as the number of quantum gate matrices will depend on the type of quantum gate and the number of qubits that the quantum gate is operating on. Generally speaking, the more qubits in the system, the more gate matrices will be needed. For example, a quantum gate on two qubits will require four gate matrices, while a quantum gate on three qubits will require nine gate matrices. Additionally, each type of quantum gate will require a different set of gate matrices, depending on the desired operation. For instance, the two-qubit CNOT gate will require two gate matrices, while the three-qubit Toffoli gate will require three gate matrices.
In sum, there is no single answer to the question of how many quantum gate matrices are there, as the answer will depend on the type of quantum gate and the number of qubits that the gate is operating on.

2-Qubit Gates Matrix Representations & Quantum Circuits, Controlled Not/CNOT, Controlled Z/CZ, SWAP
In conclusion, the world of quantum computing is still relatively new and constantly evolving. As such, the number of quantum gate matrices is not finite, and it is difficult to determine an exact count. However, researchers and scientists are continuously working to develop new and innovative quantum gate matrices that can help to improve the performance and capabilities of quantum computing.
As quantum computing continues to mature and evolve, the possibilities for the development of new quantum gate matrices are virtually limitless. As such, it is an exciting time for those in the field of quantum computing, as we continue to unlock new and innovative ways to improve the speed and efficiency of these powerful machines. With further research and development, the number of quantum gate matrices will undoubtedly continue to grow, and who knows what incredible advancements and breakthroughs we will be able to achieve in the near future.